This test uses JavaScript to generate your test and mark it. You therefore need to enable Scripting using the Preferences... item in one of the Menu items for this Browser's window. Once enabled, just Reload (Refresh) this page.

Algebra: Forming and Solving Equations
The length of a rectangular field is 10m longer than its width.
The perimeter of the field is 132m.
What is the area of the field?

The area is m2

tickcross



Worked Solution

Let the length and width of the field be L metres and W metres.
The first sentence tell us that L = W + 10
The perimeter is 2 (L + W) = 132.
Substituting for L in the perimeter equation gives:
2 (W + 10 + W) = 132
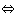<=>4 W + 20 = 132
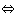<=>W = 28 metres
Putting this value into the first equation for L gives:
L = 28 + 10
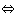<=>L = 38 metres
The area, L×W is therefore 38×28 = 1064 m2 .

© MEI Produced by Dr Ron Knott, 28 Jan 2004
tom . button [AT] mei . org . uk 		Test reference: SimFormLinEquns.html?ref=39734