Worked Solution
Dividing the equation
p x + q y + r = 0 by
q and re-arranging, we have
| y = – | | p |  | | q |
| x – | | r |  | | q |
|
| which tells us that the gradient of AB is | – | | | p |  | | q |
|
From the given coordinates, the gradient
AB here is
| By – Ay |  | | Bx – Ax |
| = | | 5 – (–6) |  | | (–1) – 4 |
| = | | – | 11 |  | | 5 |
| = – | | p |  | | q |
|
so we let
p be
11 and
q be
5The
equation of the line is therefore of the form
11 x + 5 y + r = 0Since the line goes through point
A where
x = 4 and
y = –6,
putting these values into the
equation of the line gives:
44 – 30 + r = 0 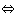 r = –14
r = –14The line
AB is therefore
11 x + 5 y – 14 = 0 or any multiple of this equation.
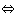 r = –14
r = –14