Worked Solution:
The differential equation is
second order,
homogeneous and has constant coefficients.
We can therefore use the
auxiliary equation:
3 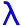 2 – 14
2 – 14 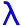 – 5 = 0
– 5 = 0It factorises:
(3 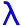 + 1) (
+ 1) (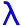 – 5) = 0
– 5) = 0| The two roots of the auxiliary equation are | | – | 1 |  | | 3 |
| and | 5 |
The
general solution is
y = A e – 1/3 z + B e5 z where A and B are arbitrary constants.
We can find
A and
B using the initial conditions:
| The general solution for y at z = 0: | | A + B = | | 7 |  | | 4 |
|
|
| Differentiating the general solution to find | | dy |  | | dz |
| at | z = 0 | : |
| | – | 1 |  | | 3 |
| A | + | 5 | B = | | 41 |  | | 12 |
|
|
| Solving these simultaneous equations: | | A = | 1 | , | B = | | 3 |  | | 4 |
|
|
| The particular solution is | y = | 1 | e – 1/3 z | + | | 3 |  | | 4 |
| e5 z |
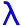 2 – 14
2 – 14 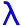 – 5 = 0
– 5 = 0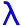 + 1) (
+ 1) (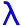 – 5) = 0
– 5) = 0