Please enable Scripting (or enable JavaScript) using Preferences... in one of the Menu items for this Browser's window.
Once enabled, just Reload (Refresh) this page to see the Test.

Differential Equations: First Order

The general solution to

4

dy du

– 3 y = 0

is

=

e

Worked Solution:

The differential equation is homogeneous, first order, constant coefficient:
so the general solution is of the form

y = A e^u   where A is an arbitrary constant.

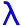The auxiliary equation to find is:

4 – 3 = 0

=

3 4

Since we have no initial or boundary conditions, the general solution is

y = A e3/4 u

© MEI Produced by Dr Ron Knott, 13 November 2006

Test reference: DEorder1.html?ref=88121