Worked Solution
Once we have the factors, we can solve the equation.
To factorize 2 x2 + 7 x + 6
Our reference formula is (px + q )( rx + s ) = prx2+ (ps + qr) x + qs
Method: find two factors of coefficient of x2 × constant term = prqs = ps × qr
that sum to the coefficient of x = ps + qr
First find the product of the x2 coefficient: 2 and the constant term: 6 which is 2×6 = 12
Now find the pairs of factors of this number:1×12, 2×6 and 3×4
and also the same pairs with both factors being negative.
Of these choose the (signed) factor pair that sums to the coefficient of x: 7 ...The pair to choose is 4, 3 because 12 = 4×3 and 4 + 3 = 7.
We can therefore rewrite our quadratic substituting 4 x + 3 x for 7 x:
2 x2 + 7 x + 6 = 2 x2 + 4 x + 3 x + 6 which enables us to factor the first two and last two terms:
2 x2 + 7 x + 6 = 2 x(x + 2) + 3(x + 2) and the bracketed term now factors out too:
2 x2 + 7 x + 6 = (2 x + 3)(x + 2)
So the solution to
2 x2 + 7 x + 6 = 0 is
2 x + 3 = 0 or
x + 2 = 0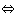 | x = | | – | 3 |  | | 2 |
| or | x = | –2 |
![tom . button [AT] mei . org . uk](sig.gif){kind=small}