Algebra: Solving Quadratic Inequalities

Worked Solution

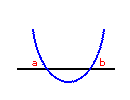First find the boundary values of the regions where the quadratic d² + d – 30 is zero:
These are d = -6 (a) and d = 5 (b)

Next determine if we want the region inside or outside the bounds.
The values of the quadratic between a and b (d > -6 and d < 5) are <0 and
the rest of the points (d < -6 or d > 5) are >0
The questions asks for d² + d – 30 > 0 and at d = 0 the quadratic is –30 i.e. <0
Since d = 0 is between –6 and 5 then we want values of d that are < –6 or >5

Finally decide if the boundary points are included or not.
The question does not include equality so we exclude a and b from the answer:

The range we require is d < -6 or d > 5