Worked Solution:
( p + a )2 = p2 + 2 a p + a2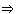 ( p + a )2 + b = p2 + 2 a p + a2 + b
( p + a )2 + b = p2 + 2 a p + a2 + bWe want to find values for
a and
b to make the line above into
p2 + 12 p – 2We already have the
p2 term so let's look at the
p term:
| For the quadratic in the question, the p coefficient is | 12 | which must be equal to the p term above: |
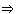 2 a = 2 a = | 12 |
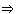 a = a = | 6 |
| a2 + b = | –2 | : The quadratic given has a constant term of | –2 |
| This must equal a2 + b and we now know the value of a = | 6 | 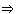 a2 = a2 = | 36 | : |
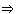 | 36 | + b = | –2 |
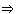 b = –38
b = –38
| y = | p2 + 12 p – 2 | = ( | p + 6 | )2 –38 |
The squared term is always positive or zero
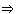 the least value of y must be –38 when the squared term is zero: ( the least value of y must be –38 when the squared term is zero: ( | p + 6 | )2 = 0 |
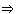 p = p = | –6 |
The turning point is therefore
( –6, –38)
 ( p + a )2 + b = p2 + 2 a p + a2 + b
( p + a )2 + b = p2 + 2 a p + a2 + b2 a =
a =
a2 =
b = –38
 the least value of y must be –38 when the squared term is zero: (
the least value of y must be –38 when the squared term is zero: (  p =
p =